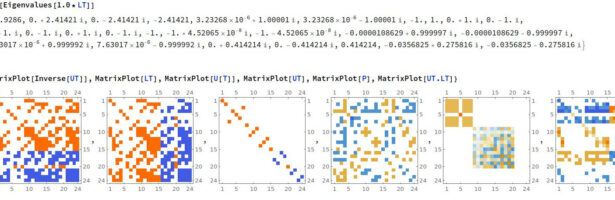
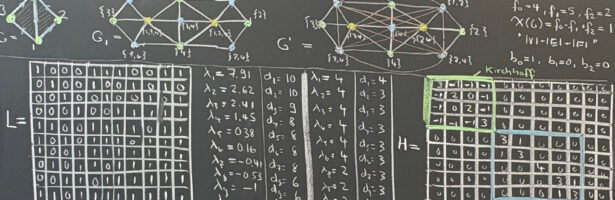
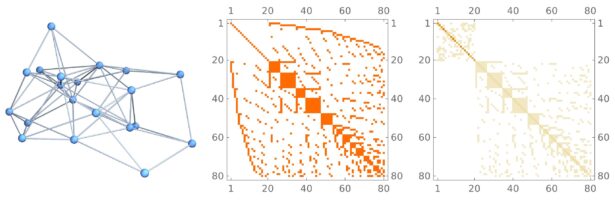
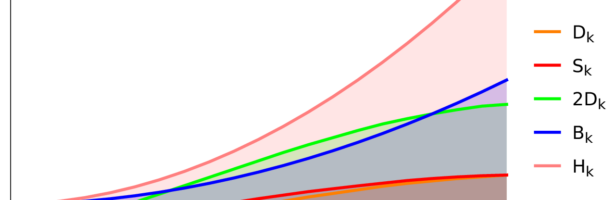
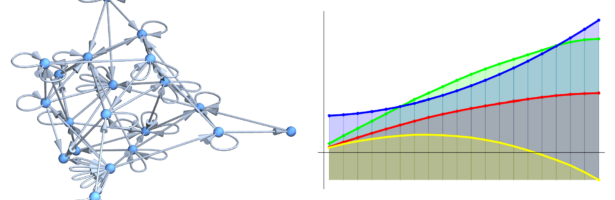
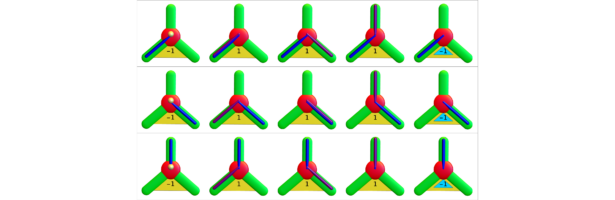
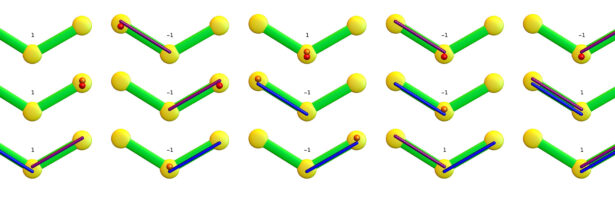
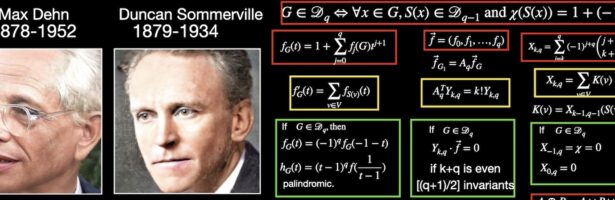
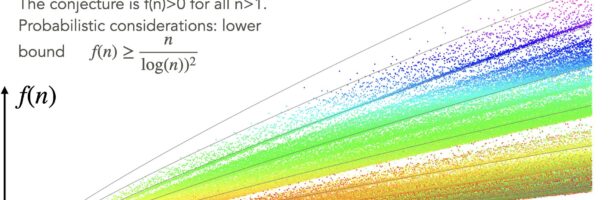
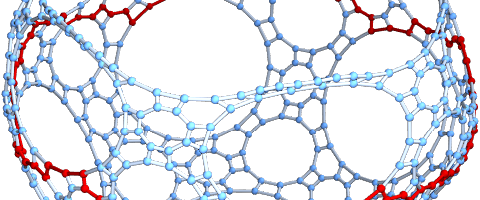
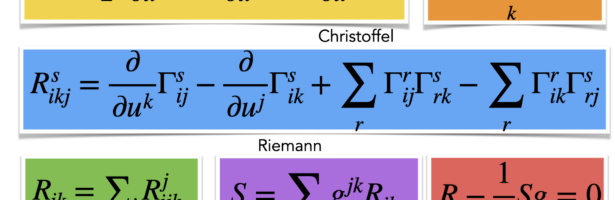
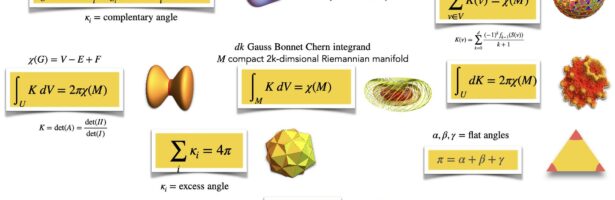
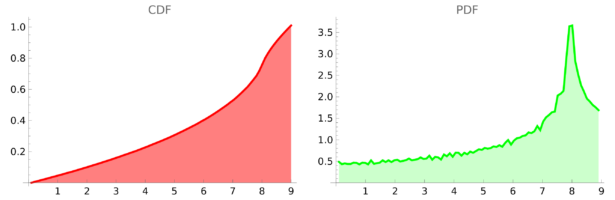
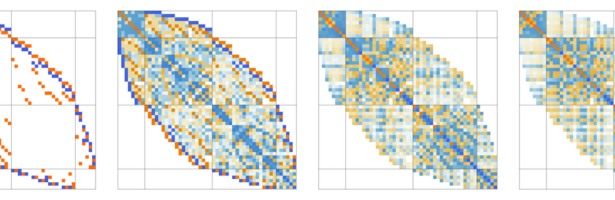
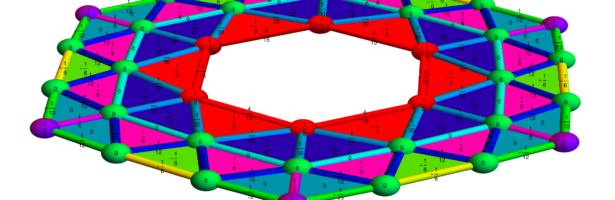
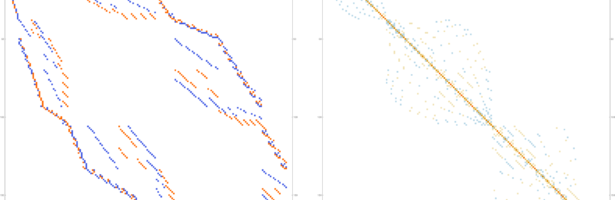
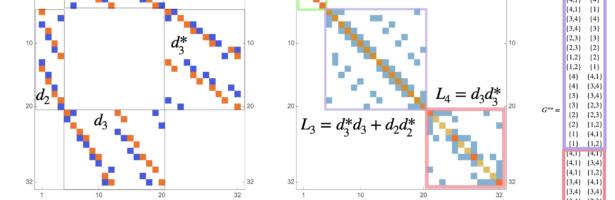
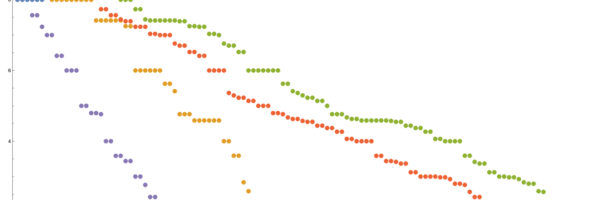
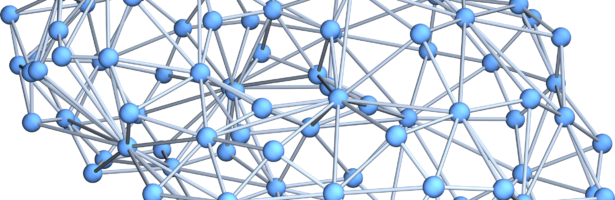
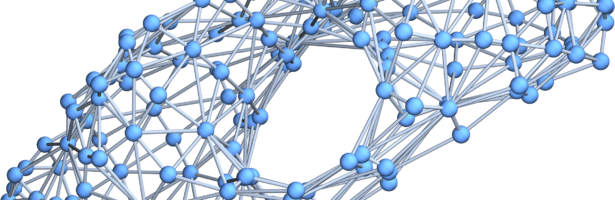
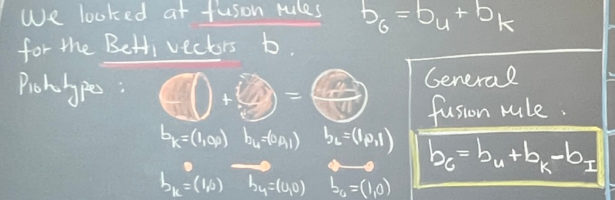Group Completing Manifolds
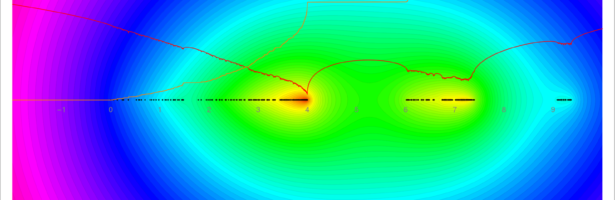
Manifolds are in the following two dimensional, compact, connected and without boundary. Their classification of these geometric objects is a classical piece of mathematics, completed in a satisfactory way in 1907 by Dehn and Heegaard: the structure is that there are two monoids. The monoid of orientable manifolds that is …