There should be an easy inductive proof of the fusion in equality , where
is the Betti vector of an open or closed set A in a simplicial complex G. Just use induction with respect to the number of elements in U. As already pointed out last time and here put again, one can now look what happens if a locally maximal simplex x is moved from K to U. There is a dichotomy both in the closed case or open case of what kernel transitions can happen. This is best seen when looking at the changes in the Dirac operators of K or U. Removing x from K just produces a row and column deletion in the Dirac operator of K. Adding x to U just produces a row and column insertion in the Dirac operator of U. We still have to write this down but I currently think this is all very simple linear algebra using the Rank-Nullity theorem. One of the nice things in this entire subject that we are in a finite combinatorial setting and have only to deal with finite matrices, no limits or approximations are needed.
In the morning before the presentation I tried to distill four important simple but powerful ideas which enter the story. There would certainly be more ,like Alexandroff’s finite topology approach from 1937 (“Discrete Raeume” was also written in German) but I focused on 4 here.
Dehn and Heegaard 1907: Looking at topology of manifolds with finitist methods without bothering to plan some sort of limit is quite bold. It is more like setting the mind into a quantum calculus framing. The structure of a finite abstract simplicial complex is the simplest geometric structure that produces really interesting mathematics. [Simpler would be just a general finite set of sets = hyper-graph but many theorems fail then]. On a simplicial complex one also has a natural finite topology, which is non-Hausdorff and so Zariski like. One could contemplate to look at other synthetic geometric frame works like incidence structures (which generalizes simplicial complexes) but that even is more complicated because the concept of an incidence framework is more complicated than just looking at one type of objects: sets. The encyclopedia entry of Dehn and Heegaard is quite amazing. It was written by two mathematicians which were not appreciated enough during their life times. Dehn and Heegaard still mix also continuum geometry in. It was not ment to be a departure from the continuum or geometric realizations. Getting rid of the continuum is not only convenient, it also opens new doors, like the Finite Alexandroff topology on a simplicial complex.
Hodge 1933: Many cohomology theories, most of them are rather abstract. A relatively elementary cohomology is obtained when we have an exterior derivative. This is historically the first. One traditionally then looks at a differential complex, chases some diagrams etc. It requires to look at equivalence classes, like cocycles modulo coboundaries or closed forms modulo exact forms. Hodge’s idea is just look at spaces of harmonic forms. This brings things into the realm of what one does in introductory linear algebra: just compute the kernel of some matrices. Given a basis in the kernel, one has concrete generators of the cohomology group. This is also relevant in physics. The Maxwell equations dF=0,d* F=0 in vacuum for example are, if (giving F=dA) and under a Coulomb gauge d*A=0 equivalent to to
, where
is the Hodge operator in space time. The d’Alembert equation HA=0 produces waves. Splitting time away gives
. Harmonic forms somehow are related to particle, Betti numbers counting their numbers. Atiyah (in a youtube video) pointed out once that he was fortunate that Hodge and Dirac who had neighboring offices did not talk much to each other, allowing him to combine these two powerful ideas “Hodge and Dirac”
Eckmann 1944: Hodge still worked in a classical framework like a Riemannian manifold or complex manifolds. The continuum is rather technical for a novice: one has to learn some tensor calculus like Cartan’s differential forms, or to use the Hodge star operator. One also needs some elliptic regularity for a proper spectral theory. I myself was not exposed to this in any course but learned it from Cycon-Froese-Kirsch and Simon in graduate school (there had been a seminar planned on Patodi’s proof of the Gauss-Bonnet-Chern theorem. The seminar never took place but I had prepared for it by reading the chapter.) Beno Eckmann is a descendent of Heinz Hopf and the doctor father of my high school teacher Roland Staerk and countless many of my Poly teachers (like Stammbach, Knus, Specker, Mislin, Jeger, Engeler). Eckmann gave popular plenary lectures for a more general audience and I went to one about Euler characteristic. A major insight is that Hodge theory does not really need the continuum. Also this is simple but powerful. I myself (before noticing the 1944 article) always felt that discrete Hodge is obvious (certainly because I had gotten that from one of Eckmann’s talks. Why is it powerful? Because it again avoids technical overhead . To much technicality can often mean that one is in the wrong frame work.
Dirac 1928: The paper “Quantum Theory of the Electron by Paul Diracbears an other simple but powerful idea. If we look at the Schroedinger equation i h u’ = L u then this is not relativistic invariant. As Poincare , Minkowski or Lorentz has seen before Einstein, the Maxwell equations are invariant under Minkowski transformations.The Maxwell equations lead to wave equations . If one could factor
this would allow to use d’Alembert from one dimension and write this as
and
. These are two Dirac equations which look like Schroedinger equations. Splitting the Laplacian requires a Clifford structure. In the one-dimensional case it is simple : use
does the job so that the Schroedinger equation for D by Taylor’s theorem produces translation. In two dimensions, Pauli matrices and in three dimensions Dirac matrices do the job. For the Hodge Laplacian d d* + d* d, no such Clifford gymnastics is needed at all. Just take D=d+d*. I think to be the first who used this operator in a combinatorial settings Example. The discrete dirac operator is featured in this ILAS conference in 2013. It played a role for the Lefschetz formula (2012) in the discrete: the super trace on cohomology is the sum over the fixed points of an endomorphism. D provides the symmetry for McKean-Singer.

Looking at the Cambridge Commons 
Flying over the roof of Austin Hall. 
Austin Hall to the right, Hemingway Gym in the center 
Photo from above Hemingway looking at the Cambridge Commons 
Rowing boat with coach 
Rowing boat on the Charles river 
Rowing boats on the Charles river 
Bird view of Harvard with Boston in the back 
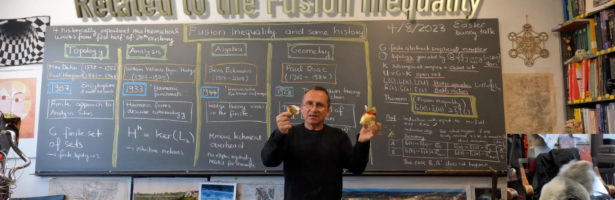
Cutting an easter bunny (a simplicial complex0 
Oliver on Easter 
Four influencal papers relevant to this work 
Inductive proof of the Fusion inequality: just move bricks from closed to open sets 
Passing by Abraham Lincoln standing on the Civil war memorial. 
American Revolution Memorial at Flagstaff Park 
diving down to the science center plaza 
Passing the tower on Harvard Hall 
Photo from above Gannett at Harvard looking into the Cambridge Commons 
Above the observatory on top of the science center 
Flying over the center for European studies 
Zooming by close to Memorial Hall 
zooming by the Harvard Memorial church 
Memorial churcdh with Boston in the background 
Passing by the entrance to the Littauer Center 
Following a seagull on the Charles river