The Maze Theorem

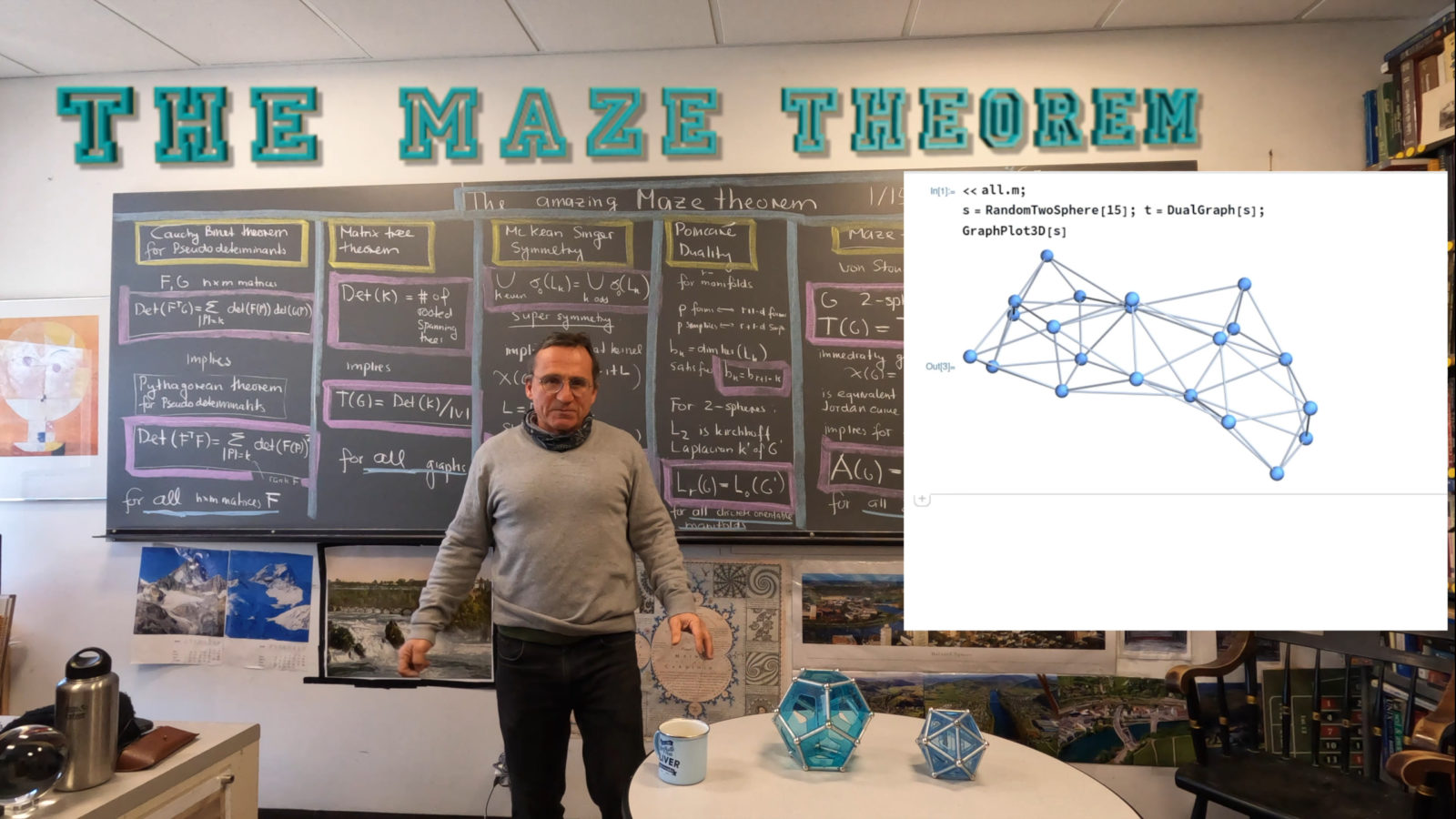
I made on January 16th a short video related Torsion and called it the Maze theorem. It is the beautiful fact already mentioned earlier here that for 2-spheres, the number of spanning trees is the same than the number of spanning trees of the dual graph. This is an old 170 year old result but rather hidden. First of all, the result holds more generally for planar graphs but for planar graphs one leaves combinatorics. Except if one uses an old result of Whitney which identifies 2-spheres as maximal planar and 3-connected graphs. The result is so much nicer to explain for 2-spheres, where no topological awkwardness comes in to define what a face is. A 2-sphere is a finite simple graph for which every unit sphere is a circular graph with 4 or more elements and which if one vertex is removed becomes a contractible graph. The Maze theorem can now be seen essentially with one picture if one accepts the discrete Jordan curve theorem assuring that on a 2-sphere, a closed simple curve of length 4 or larger has an inside and outside. The dual graph spanning tree is now just defined by the complement of the edges in the given spanning tree (maze). It is a marvelous argument due to von Staudt that this is equivalent to the Euler Gem formula as the number of edges in the maze is V-1 and the number of edges in the dual maze is F-1 and the two sets together produce all the E edges, we have (V-1) + (F-1) = E, which is the Euler Gem formula V-E+F = 2. We have seen earlier that this von Staudt argument gives together with a McKean-Singer simplification that the analytic torsion of a 2-sphere is A = V/F. A higher dimensional argument will show that in general, for arbitrary even dimensional spheres, the same formula holds where F is the number of facets, largest dimensional complete sub-graphs of the sphere. And in odd dimensions, the formula is A=V F. Having to start tomorrow in the new semester (the winter break ended too quickly), I infused some mazes also into an intro welcome lecture of a calculus course Math 22b, https://www.youtube.com/watch?v=ufLwRBoTVzU and will use mazes for the first lecture of Math E 320 (it is mentioned in the welcome lecture there: https://www.youtube.com/watch?v=58Jx2AMW5oQ. As for the winter work on analytic torsion, I decided to throw out an early draft on the subject already: as I joked several times, we never know what lies ahead and the last time I worked on analytic torsion was in the summer of 2015 which is 7 years ago. Anyway, https://people.math.harvard.edu/~knill/graphgeometry/papers/reidemeister.pdf is a first draft [PDF].